티스토리 뷰
- 항상 헷갈려서 정의...
1. 벡터란?
- 수학에서는? Vector Space의 원소가 바로 벡터
- 벡터 공간의 종류가 엄청 다양하기 때문에 물리적 직관을 함부로 적용하기 힘듬
- 함수들로 이루어진 벡터공간도 존재하며, 벡터 공간으로 이루어진 벡터 공간도 존재
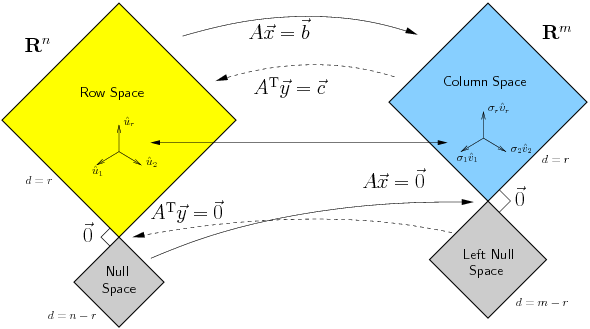
2. 백터의 내적(vector inner product)
- dot product = scalar product
- 효율
2.1 속성(property)
- Commutative
- Distributive over vector addiction
- Bilinear
- Scalar multiplication
- Orthogonal
- No cancellation
- Product Rule
2.2 Application to the law of cosines


3. 벡터의 외적
- 회전
- 벡터의 텐서곱
- 열벡터로 표현되는 두 벡터를 외적하게 되면 행렬을 얻게됨
Outer Product(외적) --> 두 벡터 간의 Tensor product
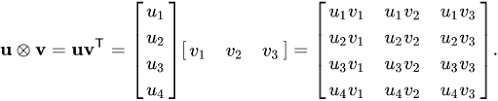
Cross Product(벡터곱 = 벡터의 외적) --> Exterior Product
- 수학에서 3차원 공간의 벡터들간의 이항 연산의 일종
- 연산의 결과가 스칼라인 스칼라곱과는 달리 연산의 결과가 벡터
- 물리학의 각운동량, 로런츠 힘 등...

1. https://www.youtube.com/watch?v=3XHWVFMR8jI
2. https://math.stackexchange.com/questions/182000/relation-between-cross-product-and-outer-product
Relation between cross-product and outer product
If inner products ($V$) are generalisations of dot products ($ \mathbb{R}^n$), then are outer products ($V$) also related to cross-products ($ \mathbb{R}^3$) in some way? A quick search reveals that
math.stackexchange.com
'인공지능 > 캐글' 카테고리의 다른 글
| pytorch에 Albumentations란? (0) | 2020.06.28 |
|---|---|
| Evaluation 종류 (추가예정) (0) | 2020.06.10 |
| Kaggle Project - Pima Indians Diabetes Database (0) | 2019.10.17 |
| kaggle을 위한 데이터 분석 [1] - 데이터 파악 (0) | 2019.10.11 |
| Kaggle Project - Predict Future Sales (0) | 2019.10.06 |
